I’m currently helping my 15-yr-old son revise for his maths GCSE, and one topic is “finding the nth term of a quadratic sequence”. I’m an ex high school maths teacher, but I had forgotten how to do this. I couldn’t find decent complex examples on either of my favourite GCSE maths revision sites (Maths Genie and BBC Bitesize), and when you’re doing the more complex examples, a step-by-step guide is really useful.
So I’m placing my notes here in case they’re any use to anyone else.
You’re aiming for a result of an2 + bn + c, but easier examples might have a solution of an2 + b, and even easier ones will just be an2.
Simplest Example (an2):
Find the nth term for the following quadratic sequence: 3, 12, 27, 48, …
First calculate the gaps between the numbers – these are 9, 15 and 21.
Then find the gaps between the gaps – these are 6 and 6. Like this:

Take that 6 and divide it by 2 (it’s easy to forget to divide by 2!), to get 3. This tells you that your final result will contain the term 3n2.
I’ve already told you that this is a simple example – we’ve reached our solution: 3n2. But you should always check your results:
| n | 1 | 2 | 3 | 4 |
| n2 | 1 | 4 | 9 | 16 |
| 3n2 | 3 | 12 | 27 | 48 |
Yup, that’s our original sequence.
More Complex Example (an2 + b):
Find the nth term for the following quadratic sequence: 1, 10, 25, 46, …
First calculate the gaps between the numbers – these are 9, 15 and 21.
Then find the gaps between the gaps – these are 6 and 6. Like this:

Take that 6 and divide it by 2 (it’s easy to forget to divide by 2!), to get 3. This tells you that your final result will contain the term 3n2.
Create a grid, which starts with your original sequence. Below that, add whatever rows you need to help you calculate 3n2.
Now, subtract 3n2 from the original sequence. So in the below grid, we subtract the fourth row from the first row, and that gives us a new sequence, which we have placed in the fifth row:
| start | 1 | 10 | 25 | 46 |
| n | 1 | 2 | 3 | 4 |
| n2 | 1 | 4 | 9 | 16 |
| 3n2 | 3 | 12 | 27 | 48 |
| start minus 3n2 | -2 | -2 | -2 | -2 |
We now have a row of constant numbers. This tells us we can reach a solution. It tells us to add -2 to 3n2, and that will be our solution: 3n2 – 2.
We can easily check this by adding up the fourth and fifth rows, which gives us the first row (the original sequence).
Most Complex Example (an2 + bn + c):
Find the nth term for the following quadratic sequence: -8, 2, 16, 34, …
First calculate the gaps between the numbers – these are 10, 14 and 18.
Then find the gaps between the gaps – these are 4 and 4. Like this:

Take that 4 and divide it by 2 (it’s easy to forget to divide by 2!), to get 2. This tells you that your final result will contain the term 2n2.
Create a grid, which starts with your original sequence. Below that, add whatever rows you need to help you calculate 2n2.
Now, subtract 2n2 from the original sequence. So in the below grid, we subtract the fourth row from the first row, and that gives us a new sequence, which we have placed in the fifth row:
| start | -8 | 2 | 16 | 34 |
| n | 1 | 2 | 3 | 4 |
| n2 | 1 | 4 | 9 | 16 |
| 2n2 | 2 | 8 | 18 | 32 |
| start minus 2n2 | -10 | -6 | -2 | 2 |
We don’t have a row of constant numbers yet, so we need to keep working. We need to look at the gaps between the numbers in our new sequence (in the bottom row of the table):

Now we have found a constant difference. This tells us that there will be a 4n in our answer. Note that this is because we have found a linear sequence. Note also that in the case of a linear sequence, we do NOT divide the number by 2.
So now we add some more rows to our grid. First we calculate 4n, and then we calculate 2n2 + 4n. Finally we subtract (2n2 + 4n) from our original sequence (subtract the 7th row from the first row):
| start | -8 | 2 | 16 | 34 |
| n | 1 | 2 | 3 | 4 |
| n2 | 1 | 4 | 9 | 16 |
| 2n2 | 2 | 8 | 18 | 32 |
| start minus 2n2 | -10 | -6 | -2 | 2 |
| 4n | 4 | 8 | 12 | 16 |
| 2n2 + 4n | 6 | 16 | 30 | 48 |
| start minus (2n2 + 4n) | -14 | -14 | -14 | -14 |
We now have a row of constant numbers. This tells us we can reach a solution. It tells us to add -14 to 2n2 + 4n, and that will be our solution: 2n2 + 4n – 14.
We can easily check this by adding up the seventh and eighth rows, which gives us the first row (the original sequence).
More worked complex examples

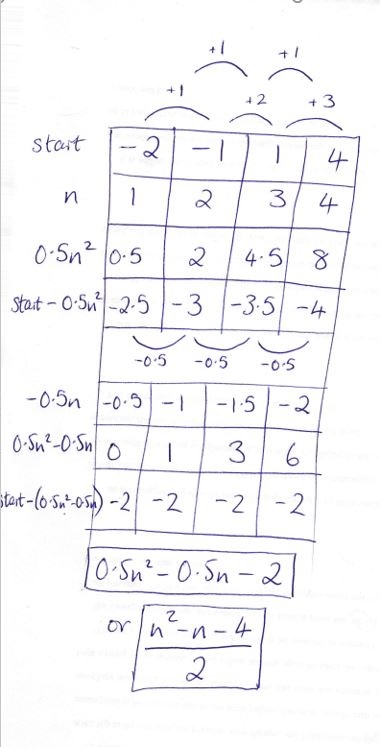
Note that in this next one there is a NEGATIVE difference between the terms of the sequence on row 5. This one can easily catch you out. Rather than thinking of the difference between the numbers, it helps to ask yourself, “how do I get from each term to the next one?” The answer in this case is, “subtract one”. This one can also look a little tricky because it contains fractional numbers, but you just follow the same rules as before:

Telling the Difference Between a Linear Sequence (an + b) and a Quadratic Sequence (an2 + bn + c).
When we calculate gaps between the numbers in the sequence, if the first level of gaps is constant, this means it is a linear sequence:

If the second layer of gaps is constant, it is a quadratic sequence:

I’ve never seen this before, thanks!
Is it somehow curve fitting, and the two differences are like the first and second derivatives (the dy in dy/dx where dx=1 because it’s discrete)?
I’m not sure what I’m talking about, nor if that question even makes sense…
I’m honestly not sure of the answer to your question, sorry! I feel like the answer is “Yes” but I would have to think about it quite hard. 🙂